Abstract. Increasing of radio-system noise immunity against spatially concentrated interference is achieved by optimizing the spatial processing which is an amplitude-phase forming on the array aperture with selection of the optimal spatial element position. To overcome the difficulties associated with increased optimal coordinate sensitivity to deviations of estimated non-stationary parameters, a regularized algorithm of robust spatial structure is used. A comparative analysis of the spatial processing efficiency in static and dynamic modes is carried out. High convergence speed of adaptive weight vector adjusting in spatially reconfigurable antenna arrays is achieved by pre-processing, which means changing spatial position of antenna array elements.

Keywords: optimization of spatial structure, adaptive signal-processing, preprocessing.

Introduction

The coordination of spatial structures of an observable field with spatial structures of radio system allows to increase interference immunity essentially [1,2]. At the same time high sensitivity of optimal spatial structures to deviations of field characteristics from the supposed ones does not allow to reach maximum interference immunity in case of non-stationary interferences, and accepted algorithms of optimization of spatial structure have low computing efficiency [3].

Grating lobes of antenna array pattern cause multiextremal criterion function with commensurable peaks [3,12], and refer a problem of synthesis optimal spatial structure to class of ill-conditioned problems as well. Strong dependence of optimal antenna element position on inaccuracy in initial data does not allow to apply directly interpolation methods for determination of antenna coordinates and leads to computing complexity increase. In previous paper regularized algorithms of spatial structure optimization for elimination of these disadvantages are offered [4]. This algorithm allows to synthesize robust spatial structures [5,6] with the size of the obtained spatial structure defined according to accepted metrics as the stabilizer is used [5]. As a result the effect of stabilization of optimal structure is found out in a static mode. It gives a possibility to raise computing efficiency by mean of interpolation methods in the case of calculation of antenna array coordinate.

Efficiency of spatial interference rejection considerably depends on properties of interference correlation matrix, particularly on its eigenvalues. For large difference of eigenvalues the convergence speed for gradient algorithms of adaptation decreases, and the sensitivity of matrix inversion result to errors of its estimation increases. This condition occurs if signal environment includes strong source of interference together with other weaker but nevertheless potent interference sources. This condition also obtains if two or more very strong interference sources arrive at the array from closely spaced directions [7].

Known methods of elimination of these drawbacks use various types of preprocessing: an accelerated gradient procedure [7], scaled conjugate gradient descent [8], cascade preprocessors with resolving the input signals into their eigenvector components. In this paper it is offered to implement preprocessing by variation of spatial structure of the receiving antenna array. Previously optimization of antenna array spatial structure was used for increase of signal-to-interference ratio [3], faster reorganization of spatial structure [9], optimization of non-linear signal processing in the presence of non-gaussian interferences [1,2].

Objective of the paper is the enhancement of adaptation speed for spatial interference compensation by means of preprocessing on the base of spatial structure optimization in antenna array.

1. Dynamic Properties of Radiosystem with Spatial Structure Optimization in Presence of Interferences
1.1 Problem Statement

The research objective is to improve interference immunity of radiosystems with optimization of spatial structure in static and dynamic operating modes under the assumption of mechanical inertance of spatial structure tuning.

The discussed radiosystem consists of an antenna array with amplitude-phase control. The sum of the determined desired signal and several point-source broadband gaussian interferences is observed on an output of each antenna array element. Interference immunity is defined as a signal-to-interference ratio on an output of spatial processing system. The spatial structure is specified by a vector of coordinates of antenna array elements . Criterion function of spatial structure optimization is accepted as a likelihood ratio . The stabilizer is a size of spatial structure [5], which is defined as a distance of given spatial structure from some mid-point of this structure. A measure of difference of one spatial structure from another is a metric in space of coordinates of antenna elements on some surface . The distance between two subsets from this set is defined on set as follows [5]:

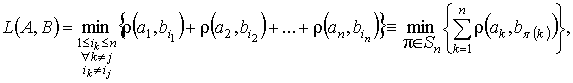
where - distance between elements of coordinate subsets , , and minimization is made on all permutations from elements of subset ; characterize a position of -th spatial sample in rectangular coordinate system. Hence a presence of distance on set transforms it into metric space. The metrics allows to solve a problem of discrimination of spatial structures .

Let an averaged likelihood ratio is a convex continuous function in some neighbourhood, which has nonempty subset of points of minimum . The stabilizer is a strongly convex continuous function which characterize degree of difference from a mid-point of spatial structure and take on form .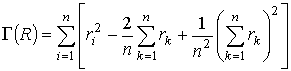

The regularized algorithm of spatial structure optimization looks as follows [5]:

(1.1)

The function is multiextremal in typical situations, therefore in a range of definition there can be some points , , and each ones has such neighbourhood that there is , where - coordinates of the local minima, and one of which is coordinate of a global minimum:

(1.2)

A search of the solution of multidimensional problem (1.2) is reduced to one-dimensional multistage problem solved by means of local extremum search methods [5,10,11]:

, (1.3)

where

is a antenna element number is a step number, is convergence factor of gradient algorithm. Thus, solving of a multiextreme problem is reduced to sequential solving local extremum problems (1.1) for all n antenna elements. Iterative procedure (1.3) repeats while value of an error not above a preset value. After that reduction twice of subarea is made, and calculations repeat while the same requirement is met.

1.2 Calculation Results

The analysis of optimization algorithm (1.1)-(1.3) is carried out by means of computing experiment for a case of the linear antenna array at number of interferences which are distributed uniformly in angular sector size =0,5 radian and located at an angle to a normal line of antenna plane. The three-element antenna array is chosen, the distance between first and last elements is fixed and is equals to 10, and the medium element is moveable.

Estimation of synthesized topology is carried out by means of analysis of occurrence frequency of distance when random initial values , , with uniform probability density function on . In the absence of regularization there are some spatial structures with commensurable frequencies of occurrence for one position of signal and interferences. The regularization factor increase to some critical value gives stability of solutions. Optimal structures slightly differ one from another when changes of interference parameters is small and thereby this structure become robust. Dependence allows to define a critical value of regularization parameter, providing stability of solution and uniqueness of optimal spatial structure for various . The size of angular sector essentially influences on distance at : increase of reduces influence of interferences on spatial structure, and reduction of increases distance and size of the antenna aperture. The reason for that is a necessity to increase angular resolution as signal source is spaced nearer to interferences sources. Thus, application of regularization provides uniqueness of solution for optimization procedure and provides its poor sensitivity to initial conditions and to parameters of interferences as well.

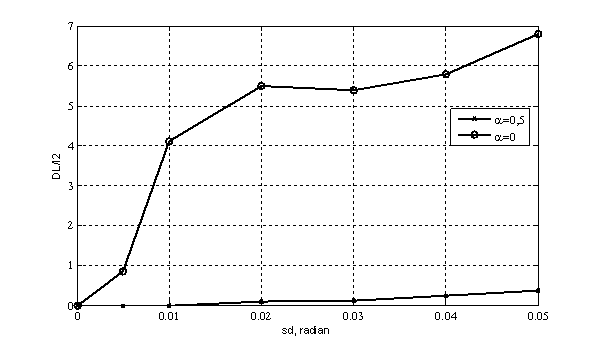
Figure 1

Regularization influence on solution sensitivity to small deviations of interference spatial position is presented on Figure 1. Dependence of a metric dispersion for accepted optimal structures on root-mean-square deviation of interferences angular position with uniform distribution shows that application of the stabilizer decreases metric dispersion in 20 … 50 times and more.

Calculation of dependence of normalized signal-to-interference ratio on regularization parameter at various positions of interference angular sector is carried out, where

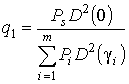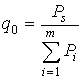
are relations of signal power coming from direction , to power of uncompensated interferences with and without taking into account the directivity of the antenna array respectively, is signal power, , are power and angular coordinate of interference, - antenna array pattern. The analysis shows that grows as increase. This fact can be explained by increase of signal and interferences spatial diversity. Efficiency gain from use of spatial structure optimization is 9,8 dB in comparison with equidistant array at the preset number of interferences and antenna elements.

Continuous dependence of optimal element coordinates after synthesis of robust spatial structures, on spatial position of a signal and interferences allows to use interpolation methods for calculation intermediate values of coordinates at rather small number of interpolation points. The optimal structure is defined at interpolation points in advance for all combinations of power values and angular coordinates of the preset number of interferences. So the definition of optimum spatial structure in real time is possible as interpolation procedures possess high computing efficiency.

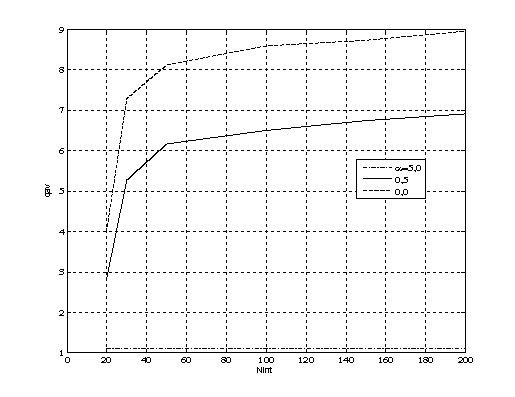
Figure 2

Dependence of signal-to-interference ratio averaged by all positions of interference sector on quantity of linear interpolation points are represented on Figure 2 both with regularization and without of this one . Interpolation is applied to position of movable antenna element at change of . Having set permissible losses for signal-to-noise ratio, it is possible to define necessary number of interpolation points for optimization procedure carrying out. So at reduction of points number to at computing complexity decrease not less than in 5 times under the set conditions of modeling, and the general losses in comparison with maximum value of signal-to-interference ratio efficiency-gain at make 1,7 dB in a static mode.

Owing to interpolation, computing efficiency strongly increases at use of two-dimensional antenna arrays, at higher number of interferences in all forward hemisphere, at various level of power.

Mechanical element moving possesses noticeable inertance which should be considered at the analysis of spatial optimization dynamic mode at not-stationary interferences. Speed of spatial structure variation depend on maximum possible change of element coordinate , expressed in wavelengths , for one time step of spatial structure optimization system, and spatial non-stationarity of interferences is set by interference angular sector moving for the same time interval.

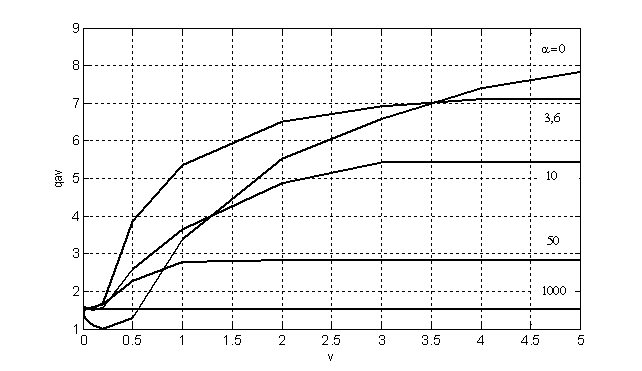
Figure 3

Research results are shown on Figure 3 in the form of dependence of signal-to-interference ratio averaged by for system with spatial structure optimization at dynamic operating mode. The analysis was carried out by modeling at various values of regularization parameter at angular speed of interferences . It is the established fact that at values of velocity parameter l, regularization at provides the maximum benefit in interference immunity up to 6 dB in comparison with a case of spatial structure optimization at a=0. Expansion of signal-to-interference ratio averaging borders and increase in interference movement velocity expands parameter area which gives additional signal-to-interference ratio gain.

2. Enhancement of Adaptation Speed In Signal Processing System With Optimization Of Spatial Structure

2.1 System Model and Signal Processing

Let us consider an -element planar antenna array with axial coordinates of elements . The array is divided on two sub-arrays of and elements respectively, . The first sub-array of elements is intended for source direction finding and forms the main pattern. The second sub-array of elements is intended for spatial interference compensation and contains elements which provide the adaptive adjustment of amplitude and phases of interferences. Coordinates of elements can be variable for obtaining of the best interference immunity.

Interference environment is set by point sources with power , allocated in the directions set by angles of azimuth and by angles of elevation , . Vector of complex observable signal represents a set of complex envelopes for signal and interference on outputs of antenna elements at the same point of time:

. (2.1)

Matrix of spatial correlation for interferences on outputs of antenna elements:

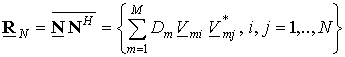, (2.2)

where 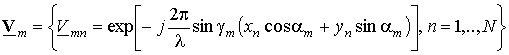- interference source position vector, which depends on antenna element coordinates, sign denotes Hermitian conjugation, - wavelength.

It is possible to represent signals on outputs of first and second sub-arrays as follows:

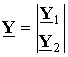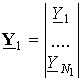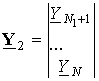, , . (2.3)

In this case correlation matrix has a block structure:

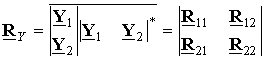and optimal weight processing vector minimizes the power of uncompensated residuals of interferences at =const [7].

Optimization of spatial structure of the second sub-array is carried out by criterion (2.4) of maximum ratio of minimum and maximum eigenvalues of matrix , defining speed and stability of adaptation process:

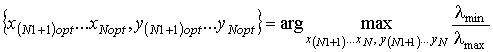(2.4)

The criterion of maximum eigenvalues ratio allows to raise efficiency of interference rejection by solving the following problems:

- enhancement of convergence speed for the adaptive weight adjustment using gradient algorithms,

- reduction of correlation matrix inversion error,

- increase of suppression ratio by more exact nulling on antenna pattern.

Figure 4.Spatial adaptive processing with spatial structure optimization

2.2 Numerical Analysis

Let us carry out the statistical modeling of preprocessing with optimization of spatial structure and processes in system with the adaptive spatial signal processing at various parameters of the antenna array ( Fig. 4).

For calculation of optimal vector value , where - dispersion of estimation error of the desired signal, we use a steepest descent method [7]:

where - convergence factor, - interferences suppression error.

An example of convergence result for weight adjustment process in case of presence and absence of preprocessing with optimization of space structure (2.4) is represented in Fig. 5. Modeling conditions: interference-to-noise power ratio =20, =0,001, =2, , interferences angles of arrival =25^о, =50^о, aperture size of array.

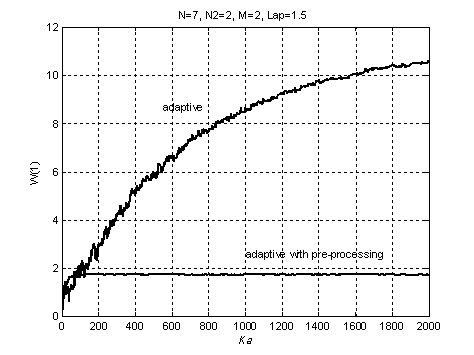
Figure 5. Adjustment of weight at adaptation with preprocessing

Fig.6 illustrates the same situation as the Fig.5 from the point of antenna pattern nulling for adaptation with the preprocessor and without it. In this case the number of adaptation steps =100 corresponds to time of the preprocessing, which increase speed of convergence. Thus adaptive antenna pattern with the preprocessing practically coincides with the optimal one.

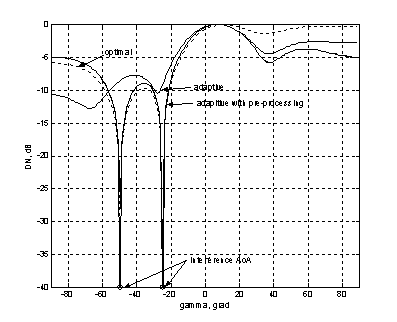
Figure 6. Antenna pattern nulling and adaptation with preprocessing

Figure 7. Dependence of minimum eigenvalues ratio on aperture size

The adaptation speed gain is illustrated by dependences of minimum optimal eigenvalues to original eigenvalues ratio

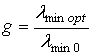
on aperture size of original uniformly spaced antenna array, Fig. 7. It is necessary to mark that maximum gain of adaptation speed is reached in the cases of small sizes of the antenna array aperture.

Conclusion

Thus regularized algorithm of spatial structure optimization provides poor sensitivity signal-to-interference ratio to deviations from suppositions, and the introduced stabilizer limits the size of spatial structure. It is shown that optimal spatial structures, obtained as a result of regularization, possess stability both to deviations of antenna element initial positions and to change of signal and interference positions. These properties of optimal structures allow to solve a number of technical problems facing before developers of radiosystems. For example, for increase of computing efficiency there is possibility to use interpolation of optimal structures for the values calculated in advance and stored in database. Other advantage of regularized spatial structure optimization algorithms is continuous dependence of antenna element coordinates on parameters of interferences that allows to lower requirements to velocity of reorganization and, thus, to establish optimum coordinates of elements more precisely, to increase signal-to-interference ratio in comparison with absence of regularization.

Introduction

1.2 Calculation Results

Conclusion

References